Area of a Triangle Calculator - Find Triangle Area
Select a calculation method and enter the values required.
Result:
Our Triangle Area Calculator is a comprehensive tool designed to calculate the area of any triangle using multiple proven methods. Whether you're a student learning geometry, an engineer working on structural designs, or a professional needing precise area calculations, this calculator provides instant, accurate results with detailed step-by-step solutions for various triangle configurations.
Understanding triangle area calculations is fundamental in mathematics and has countless practical applications in construction, engineering, architecture, landscaping, and design. From calculating the area of triangular roof sections to determining material requirements for triangular garden beds, triangle area calculations are essential skills in both academic and professional settings.
Our calculator supports multiple calculation methods to accommodate different scenarios: base and height measurements, all three sides (Heron's formula), two sides with included angle (SAS), two angles with included side (ASA), and special cases like equilateral, isosceles, and right triangles. Each method is optimized for specific types of triangle problems you might encounter.
How to Use the Triangle Area Calculator
Our versatile calculator makes triangle area calculations effortless:
- Select Method: Choose from multiple calculation methods based on available information
- Enter Values: Input the required measurements (sides, angles, base, height)
- Calculate: Click the "Calculate" button for instant results
- Comprehensive Results: View area calculations with step-by-step solutions
- Multiple Formats: See results in different units and decimal precision
Understanding Triangle Area: Fundamental Concepts
Triangle area represents the amount of two-dimensional space enclosed within the three sides of a triangle. It's measured in square units and is fundamental to geometry, trigonometry, and practical applications in construction, design, and engineering.
Essential Triangle Components
- Base: Any side of the triangle can serve as the base
- Height (Altitude): The perpendicular distance from the base to the opposite vertex
- Sides: The three line segments that form the triangle's boundaries
- Vertices: The three corner points where sides meet
- Angles: The three interior angles that sum to 180°
Triangle Area Calculation Methods
Different methods are used depending on the available information about the triangle:
Method 1: Base and Height Formula
A = (1/2) × base × height
This is the most fundamental formula, where:
- A = Area of the triangle
- Base = Length of any side chosen as the base
- Height = Perpendicular distance from base to opposite vertex
Example 1: Base and Height Method
Given: base = 12 cm, height = 8 cm
Given: base = 12 cm, height = 8 cm
A = (1/2) × 12 × 8A = (1/2) × 96A = 48 cm²
Method 2: Heron's Formula (Three Sides)
A = √[s(s-a)(s-b)(s-c)]
Where s = (a+b+c)/2 is the semi-perimeter:
- s = Semi-perimeter
- a, b, c = Lengths of the three sides
Example 2: Heron's Formula Method
Given: sides a = 3, b = 4, c = 5
Given: sides a = 3, b = 4, c = 5
s = (3 + 4 + 5)/2 = 6A = √[6(6-3)(6-4)(6-5)]A = √[6 × 3 × 2 × 1] = √36 = 6
Method 3: Two Sides and Included Angle (SAS)
A = (1/2) × a × b × sin(C)
When you know two sides and the angle between them:
- a, b = Lengths of two sides
- C = Angle between the two known sides
Example 3: SAS Method
Given: a = 8, b = 6, angle C = 30°
Given: a = 8, b = 6, angle C = 30°
A = (1/2) × 8 × 6 × sin(30°)A = (1/2) × 48 × 0.5A = 12
Method 4: Two Angles and Included Side (ASA)
A = (a² × sin(B) × sin(C)) / (2 × sin(A))
When you know two angles and the side between them:
- a = Length of the known side
- B, C = The two known angles
- A = The third angle (A = 180° - B - C)
Special Triangle Types and Formulas
Equilateral Triangle
A = (√3/4) × s²
All sides equal, all angles 60°:
- s = Length of any side
- √3 ≈ 1.732
Isosceles Triangle
Two equal sides and two equal angles. Can use any standard formula, but base-height is often most convenient.
Right Triangle
A = (1/2) × leg₁ × leg₂
One 90° angle, where legs are the two perpendicular sides:
- leg₁, leg₂ = The two sides forming the right angle
- The legs serve as base and height
Scalene Triangle
All sides different lengths. Heron's formula is often most practical when all sides are known.
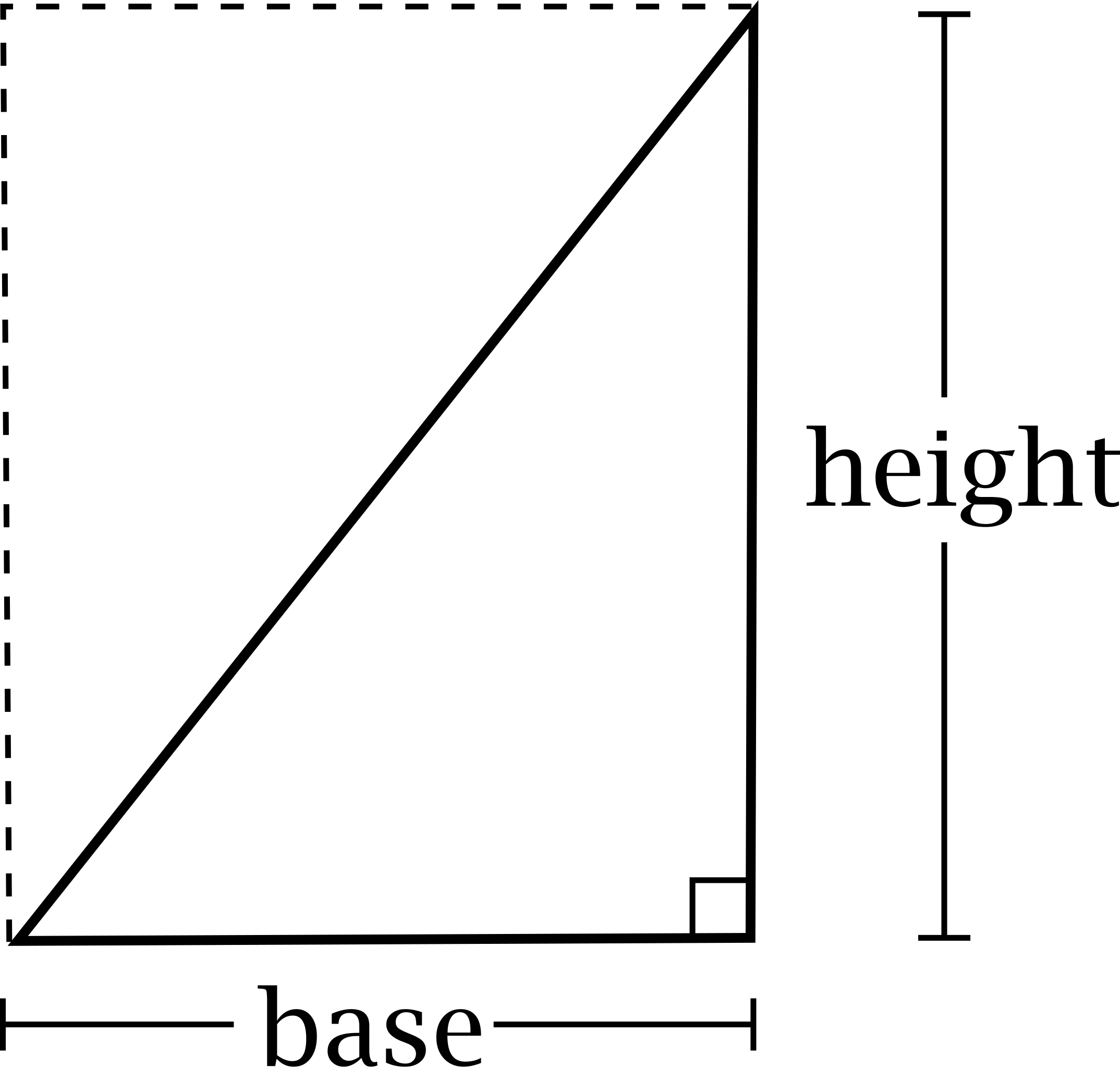
Real-World Applications of Triangle Area Calculations
Construction and Architecture
- Roofing: Calculating triangular roof section areas for material requirements
- Structural Design: Determining load-bearing capacity of triangular trusses
- Land Surveying: Measuring triangular plots and property boundaries
- Foundation Work: Planning triangular foundation elements
Engineering and Manufacturing
- Mechanical Design: Calculating areas of triangular components and parts
- Aerospace: Wing section analysis and aerodynamic calculations
- Civil Engineering: Bridge truss analysis and structural calculations
- Material Sciences: Stress analysis on triangular sections
Art, Design, and Landscaping
- Garden Planning: Calculating areas of triangular garden beds and paths
- Interior Design: Planning triangular spaces and decorative elements
- Textile Arts: Pattern making and fabric requirements for triangular shapes
- Graphic Design: Layout planning and geometric design elements
Navigation and Geography
- Triangulation: GPS and surveying techniques using triangle calculations
- Cartography: Map making and area measurements
- Marine Navigation: Course plotting and position fixing
- Astronomy: Celestial navigation and distance calculations
Advanced Triangle Area Concepts
Coordinate Geometry Method
For triangles defined by coordinate points (x₁,y₁), (x₂,y₂), (x₃,y₃):
A = (1/2)|x₁(y₂-y₃) + x₂(y₃-y₁) + x₃(y₁-y₂)|
Vector Cross Product Method
Using vectors for triangle vertices, area equals half the magnitude of the cross product.
Integration Applications
Triangle areas are fundamental in calculus for approximating areas under curves using triangulation methods.
Common Errors and How to Avoid Them
- Height Confusion: Ensure height is perpendicular to the chosen base
- Unit Inconsistency: Keep all measurements in the same units
- Angle Units: Verify angles are in correct units (degrees vs. radians)
- Triangle Inequality: Ensure the sum of any two sides exceeds the third
- Formula Selection: Choose the appropriate formula based on available information
Tips for Accurate Triangle Area Calculations
- Verify Triangle Validity: Check that given measurements can form a valid triangle
- Choose Optimal Method: Use the simplest applicable formula for your data
- Double-Check Calculations: Verify results using alternative methods when possible
- Consider Precision: Use appropriate decimal places for your application
- Understand Context: Consider real-world constraints and measurement accuracy
Practice Problems with Solutions
Problem: A triangular garden bed has a base of 15 feet and a height of 10 feet. What is its area?
Solution:
Given: base = 15 ft, height = 10 ft
Formula: A = (1/2) × base × height
Calculation: A = (1/2) × 15 × 10 = (1/2) × 150 = 75 square feet
Answer: The garden bed has an area of 75 square feet.
Problem: A triangle has sides of length 5 cm, 12 cm, and 13 cm. Find its area using Heron's formula.
Solution:
Given: a = 5, b = 12, c = 13
Semi-perimeter: s = (5 + 12 + 13)/2 = 15
Formula: A = √[s(s-a)(s-b)(s-c)]
Calculation: A = √[15(15-5)(15-12)(15-13)] = √[15 × 10 × 3 × 2] = √900 = 30
Answer: The triangle has an area of 30 square cm.
Problem: An equilateral triangle has sides of length 8 inches. What is its area?
Solution:
Given: side length s = 8 inches
Formula: A = (√3/4) × s²
Calculation: A = (√3/4) × 8² = (1.732/4) × 64 = 0.433 × 64 = 27.71 square inches
Answer: The equilateral triangle has an area of approximately 27.71 square inches.
Problem: A triangle has two sides of 7 cm and 9 cm with a 60° angle between them. Find the area.
Solution:
Given: a = 7 cm, b = 9 cm, angle C = 60°
Formula: A = (1/2) × a × b × sin(C)
Calculation: A = (1/2) × 7 × 9 × sin(60°) = (1/2) × 63 × 0.866 = 27.28 square cm
Answer: The triangle has an area of approximately 27.28 square cm.
Other Similar Calculators
Check out other calculators that are similar to this one.
Frequently Asked Questions
The basic formula for triangle area is A = (1/2) × base × height. You can also use Heron's formula with three sides, or trigonometric formulas when you know angles. Choose the method based on the information you have.
Heron's formula calculates triangle area using all three sides: A = √[s(s-a)(s-b)(s-c)], where s = (a+b+c)/2 is the semi-perimeter and a, b, c are the side lengths. This method is useful when you know all three sides but not the height.
When you know two sides and the included angle, use A = (1/2) × a × b × sin(C), where a and b are the sides and C is the angle between them. This is called the SAS (Side-Angle-Side) method.
Equilateral triangles (all sides equal) use A = (√3/4) × s². Right triangles can use A = (1/2) × leg₁ × leg₂. Isosceles triangles (two equal sides) and scalene triangles (all different sides) can use any standard formula. All triangles can use the basic A = (1/2) × base × height formula.
The formula is multiplied by 1/2 because a triangle is exactly half of a rectangle with the same base and height. When you multiply base × height, you get the area of the full rectangle, so dividing by 2 gives you the area of the triangle (half the rectangle).
Yes, you can use any units (inches, feet, centimeters, meters, etc.) as long as all measurements use the same units. The area will be expressed in square units (e.g., if measurements are in feet, the area will be in square feet).
If you don't know the height, use alternative methods: Heron's formula if you know all three sides, the SAS method if you know two sides and the included angle, or the ASA method if you know two angles and the included side. You can also calculate the height using trigonometry if you have other measurements.
Verify your calculation by using a different method if possible. For example, if you used base-height, try Heron's formula if you know all sides. Check that your triangle satisfies the triangle inequality (sum of any two sides > third side), and ensure your result makes sense given the triangle's dimensions.
Key Takeaways
- Basic Formula: A = (1/2) × base × height works for any triangle
- Heron's Formula: A = √[s(s-a)(s-b)(s-c)] when you know all three sides
- SAS Method: A = (1/2) × a × b × sin(C) for two sides and included angle
- Special Cases: Equilateral triangles use A = (√3/4) × s²
- Unit Consistency: Use the same units for all measurements
- Method Selection: Choose the formula based on available information
- Real-World Applications: Essential for construction, design, and engineering projects
Find Calculator
Popular Calculators
Other Calculators
